
Navigating the challenges of the Primary School Leaving Examination (PSLE) Math paper, students often face a variety of complex questions that test their problem-solving skills. Particularly in Paper Two, the problem sums can be daunting, requiring students to apply multiple Mathematical concepts through a series of steps. But while these questions often require more than just straightforward calculations, the strategic methods to tackle them tend to remain constant, offering a reliable framework for students.
One such effective strategy to address these multifaceted questions is the Gap and Difference method.
Understanding the Gap & Difference Method
But first, what is the Gap and Difference method? Understanding the meaning of this method is crucial, especially as it appears frequently in challenging problem sums designed to test a student’s logical and Mathematical reasoning.
The Gap and Difference method, often referred to as the Excess and Shortage method, addresses some of the trickier aspects of Primary School Math. This method revolves primarily around two pivotal elements that indicate its use:
- “If” Scenarios:These are word problems that utilise conditional statements—typically starting with “if” or “when”—to set up hypothetical situations that challenge students to think critically about the implications of changes in variables.
- Excess or Shortage: These problems specifically deal with quantities that are either too much (excess) or too little (shortage) compared to a required norm.
Visual representations such as models also play a critical role in this method, helping students visualise the relationship between different quantities and understand where the ‘gap’ or ‘difference’ lies.
A typical Gap and Difference problem might also present two scenarios with varying conditions, often marked by the presence of the word “if” twice within the setup—leading to what some educators might call ‘Double-If’ questions. These scenarios might depict:
- Excess in both conditions,
- Shortage in both conditions,
- One condition leading to an excess and the other to a shortage.
The key to mastering these problems lies in focusing on the quantity that differs between the scenarios, identifying the constant difference, and applying it to find the solution.
Question #1
For our first practical application of the Gap & Difference Method, let’s consider the following scenario:
During the Lunar New Year celebration, Uncle Chen prepared red packets for the children in his extended family. If he gives $5 to each child, he would be short of $15. However, if he gives $3 to each child, he would be short of just $3. How many children are there?
Step-by-Step Solution:
Step 1: Identifying Keywords
In this problem, the keywords are “short of” and the numbers associated with each scenario. These phrases and numbers set the conditions for applying the Gap & Difference Method.
Step 2: Model Drawing and Solution Using the Gap & Difference Method:
Draw models for each scenario. Label the total amount of money Uncle Chen initially planned to distribute and show the shortage for each distribution.
For $5 per child: Short by $15.
For $3 per child: Short by $3.

Step 3: Calculate the Gap
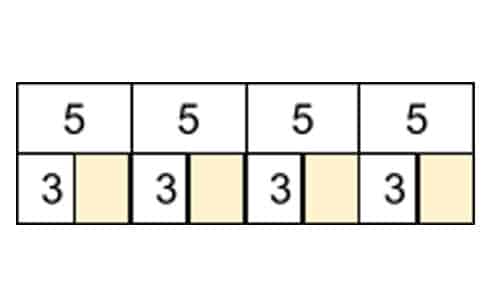
The difference in the amount given per child between the two scenarios is $5 – $3 = $2 (the value of each gap)
The shortage difference between the two scenarios is $15 – $3 = $12
Step 4: Solve for the Number of Children
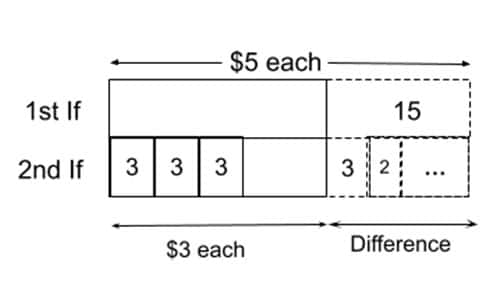
Now, let’s determine the number of gaps. Since each gap represents a value of 2, how many times do we need to add 2 to reach the difference of 12?
Each child accounts for a $2 difference in shortfall, divide the total difference in shortage by the difference per child: 12 ÷ 2 = 6
Step 5: Confirm the Calculation
With 6 children, giving $5 each requires $30, and Uncle Chen would be $15 short, indicating he has $15.
Giving $3 each requires $18, confirming the $3 shortage with $15 on hand.
Question #2
Consider a situation where two siblings, Marcus and Emily, receive an identical weekly allowance from their parents. Marcus spends $18 daily, while Emily spends $23. When Marcus has $90 left, Emily has only $25 remaining. How much money did Emily initially receive?
Step-by-Step Solution:
Step 1: Identify the Daily Spending Difference
Calculate the daily spending gap between Marcus and Emily.
Difference per day → $23 – $18 = $5
Step 2: Calculate the Total Remaining Funds Difference
Determine the difference in the remaining funds between the two.
Remaining funds difference → $90 – $25 = $65
Step 3: Determine the Duration of Spending
Divide the total funds difference by the daily spending difference to find the number of days they have been spending their allowance.
Number of days → $65 ÷ $5 = 13 days
Step 4: Compute Emily’s Total Expenditure
Calculate the total amount Emily spent over 13 days.
Total spent → 13 days × $23 = $299
Step 5: Add Back Emily’s Remaining Funds
Add the amount left to find out the initial amount Emily received.
Initial amount → $299 + $25 = $324
Variations and Challenges
When tackling Gap and Difference problems, however, students must still be adept at interpreting various problem phrasings and extracting hidden information. This is because problems may use different terms, such as “leftover” instead of “excess,” or “when” in place of “if,” which can subtly change the approach needed.
Additionally, some problems may not explicitly state the excess or shortage, requiring students to infer these values through calculations. This makes consistent practice with a diverse set of problems crucial. This exposure helps students recognise different scenarios and enhances their confidence in applying the Gap & Difference method effectively across various contexts.
Mastering P5 Math with Expert Guidance
Unlock your child’s potential in Math with our expert P5 Math tuition services at TLS Tutorials. Specialising in Primary School Math tuition, we equip students with the skills to tackle P5 Math topics and questions, including the intricate Gap & Difference method. Our personalised approach ensures that each student grasps the nuances of the subject, paving the way for academic excellence. Join us at our tuition centre for Primary School Math and watch your child thrive in P5 Math.
